In the standard notation, the area of the euclidean triangle ABC can be written as
½absin(C) or as ½ahA, where hA is the length of the altitude from A. Indeed, using
the observation that each represents the area, ½absin(C) = ½bcsin(A) = ½casin(B),
and we get a nice proof of the sine rule. The key to the proof is that these values
are equal. The fact that they represent the euclidean area is incidental. Our proof of
the sine rule in hyperbolic geometry uses the invariant Δ in a similar way.
If we recall our earlier notation for a hyperbolic triangle ABC -
a = d(B,C), b = d(C,A), c = d(A,B),
α = cosh(a), β = cosh(b), γ = cosh(c).
Then we have
sinh(b)sinh(c)sin(A) = sinh(a)sinh(b)sin(C) = sinh(a)sinh(c)sin(B) = Δ,
where Δ is the positive root of 1 - α2 - β2 - γ2 + 2αβγ.
Thus the expression sinh(x)sinh(y)sin(Z), where x, y are the hyperbolic lengths of the
sides meeting at vertex Z, is independent of Z. Note that it can be made arbitrarily
large by increasing x, keeping y and Z fixed. Thus it is not the hyperbolic area since
a hyperbolic triangle has area less than π.
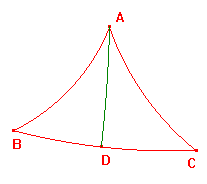
Th sketch on the right shows a hyperbolic triangle ABC and the altitude AD at the
vertex A. The triangle ABD has a right angle at D, so we an apply the sine formula
to get sin(B) = sinh(hA)/sinh(c), where hA = d(A,D), the length of the altitude.
From the formulae above, Δ = sinh(a)sinh(c)sin(B), so we then obtain
Δ = sinh(a)sinh(hA). Again by the symmetry of Δ in a,b,c we get the result
sinh(a)sinh(hA) = sinh(b)sinh(hB) = sinh(c)sinh(hC) = Δ.
We also have expressions for the length of the altitudes e.g. sinh(hA) = Δ/sinh(a).
Now we have six expressions for Δ, each of which is an analogue of a euclidean
formula for the area of a triangle. We describe them as analogues since they fit
some patterns which the reader may alrady have noted:
when we try to prove a hyperbolic version of a euclidean result, then
(1) euclidean lengths are replaced by hyperbolic functions of the hyperbolic lengths,
(2) if the euclidean result has a2, the hyperbolic one usually has cosh(a),
(3) if the euclidean result has a2+b2, the hyperbolic one usually has cosh(a)cosh(b),
(4) if the euclidean result has ab, then the hyperbolic version has sinh(a)sinh(b),
(5) constant multipliers (such as ½) tend to disappear.
We offer no serious attempt to justify these phenomena. We merely observe that
almost all of our results go back to the cosine rule, and that the observations are
valid for this.