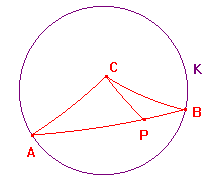
Suppose that K is a hyperbolic circle with centre C and radius r,
and that the chord AB of K passes through the fixed point P.
Let d(P,A) = a, d(P,B) = b and d(P,C) = c. Then
sinh(a+b)/(sinh(a)+sinh(b)) = cosh(r)/cosh(c) if P is inside K,
sinh(a+b)/(sinh(a)+sinh(b)) = 1 if P is on K,
sinh(a+b)/(sinh(a)+sinh(b)) = cosh(c)/cosh(r) if P is outside K.
Suppose first that P is inside K.
As CA, CB are radii, d(C,A) = d(C,B) = r.
Apply the Cosine Rule to the hyperbolic triangles ACP, BCP,
cos(<APC) = (cosh(r)cosh(a)-cosh(c))/sinh(r)sinh(a),
cos(<BPC) = (cosh(r)cosh(b)-cosh(c))/sinh(r)sinh(b).
But cos(<APC) = - cos(<BPC), so that
sinh(b)(cosh(c)cosh(a)-cosh(r)) = -sinh(a)(cosh(c)cosh(b)-cosh(r)).
cosh(c)(sinh(b)cosh(a)+sinh(a)cosh(b))= cosh(r)(sinh(a)+sinh(b)),
so that sinh(a+b)/(sinh(a)+sinh(b)) = cosh(r)/cosh(c).
If P is on K, then P = A or B. We may as well assume P = A.
Then a = 0, and c = r. sinh(a+b)/(sinh(a)+sinh(b)) = 1, as required.
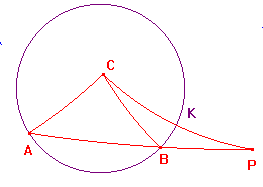
The proof for P outside K is very similar to the first part,
except that, in applying the Cosine Rule, the roles of r and c are
reversed. See the picture on the right. The result follows.