
Ideal points
The properties of incidence in the euclidean plane show a lack of symmetry,for while two distinct points always define a line, two distinct lines
either define a point or are parallel.
To achieve symmetry, we introduce additional points, called
ideal points for the plane.
Note that these are purely abstractions, they do not correspond to points in a picture.
It may be useful to think of the plane viewed at an angle,
then the ideal line corresponds to the horizon.
Definitions
The ideal line for the euclidean plane E is the set
{â : a is a direction in E}.
The elements of the set are the ideal points for E.
The extended plane Ê
consists of E, together with the ideal points for E.
A direction in E may be defined by a line through O, or as a class of parallel lines.
We must now define lines and incidence in Ê.
Definitions
If L is a line on E, then the extended line, L+,
consists of L, together with
the ideal point associated with the direction of L.
The lines of Ê are the ideal line and the extended lines
L+ for L on E.
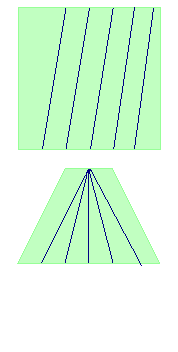
- Two points P, Q in Ê.
- P, Q on E. Then the points define a unique line L on E, and hence a unique line L+ on Ê.
- P on E, Q ideal. Let L be the line on E through P
in the direction associated with Q.
Then L+ is the only line on Ê containing both P and Q. - P, Q ideal. Then the only line of Ê containing both is the ideal line.
- Two lines L, N on Ê.
- L=K+, N=M+ for some K, M on E.
If K and M are parallel, then L and N meet only in the ideal point for their common direction.
Otherwise, K and M intersect at a point P of E. Then as L and N
have different ideal points (directions), they meet only at P. - L=K+, N is the ideal line. Then L has just one ideal point, so meets N only once.
- L=K+, N=M+ for some K, M on E.
| Two points define a line |
|
w w Two lines define a point |