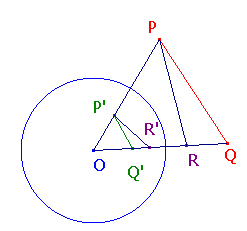
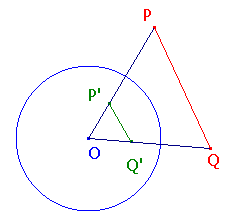
Suppose that C is a circle with centre O, and that P and Q are points other than O.
Let P and Q have inverses P' and Q' with respect to C.
Then <QPO = <OQ'P' (in magnitude and sense)
Proof
Let r denote the radius of C.
As P and P' are inverse, OP.OP' = r2.
As Q and Q' are inverse, OQ.OQ' = r2.
Thus, OP.OP' = OQ.OQ', so OP/OQ = OQ'/OP'.
Hence, triangles OPQ and OQ'P' are similar. (Note the order of the vertices!)
It follows that the given angles are equal in magnitude and sense.
Suppose that P, Q and C are as in the theorem, and that R lies on the line OQ.
Let R' be the inverse of R with respect to C.
Then <QPR = -<Q'P'R' (in magnitude and sense)
Proof
| <QPR | =<QPO - <RPO | |
| =<OQ'P' - <OR'P' | by the Theorem | |
| =<R'P'Q' | by the exterior angle property for triangle P'Q'R' | |
| =-<Q'P'R' |