with vertex N from the sphere S to the plane P.
Lemma
If the circle C on S passes through N, then the projection p(C)
is parallel to the tangent to C at N.
Proof
We know from Theorem 1 that L = p(C) is a line.
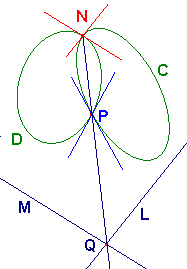
The picture is symmetric about NP, the diameter of C through N,
Suppose that C is the intersection of S with the plane F.
Now, L, C and T, the tangent to C at N all lie on F.
Thus we need only consider this plane.
so L and T are perpendicular to this diameter, and hence parallel.

Theorem 2
Stereographic projection preserves angles.
Since the angle between curves on P is defined in terms of tangents,
it is enough to consider the case of two lines L and M on P,
meeting at Q with angle a.
Suppose that these correspond to the circles C and D on S.
They meet at P = p-1(Q).
Since L and M are lines, C and D also meet in N.
By the Lemma, the tangents to C and D at N are parallel to L and M.
Thus, the angle between C and D at N is also a.
By an earlier remark, the angle beween two circles at both
intersections
are equal,
so the angle between C and D at P
is also a.